Dihedral Angle Restraints
This section shows how to restrain dihedral angles to particular values.
The functional form of the effective
energy
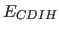
is given by
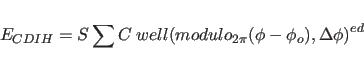 |
(7.3) |
where the sum extends over all restrained dihedral
angles and
the square-well potential
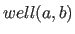
is given by
 |
(7.4) |
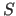
is an overall weight factor.
The constant
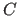
, the angle range
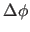
,
the angle centroid
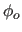
, and the exponent
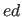
are specified
in the restraints dihedral statement. The dihedral
angle can be defined by a set of four atoms independent of
the molecular structure. In particular, the four atoms do not
have to be connected to each other. Each atom is selected
by a separate selection statement. The dihedral angle involving
four atoms i,j,k,l
is defined as the angle between two planes, where the first
plane is made by atoms i,j,k and the other plane
is made by atoms j,k,l.
Subsections
Xplor-NIH 2025-03-21