For POTEntial=3DPO, a restraining function for 3D NOE-NOE
experiments is used.
The intensity of a 3D NOE-NOE crosspeak,
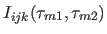
,
between spins
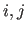
, and
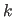
, is
proportional to the product of the individual NOE transfer
efficiencies of each mixing time:
![\begin{displaymath}
I_{ijk}(\tau_{m1},\tau_{m2}) = c[exp(-{\bf R}\tau_{m2})]_{ij}
[\exp(-{\bf R} \tau_{m1})]_{jk}A_{kk}(0),
\end{displaymath}](img664.png) |
(20.14) |
where
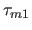
is the first and

the second mixing time,
R the cross-relaxation matrix,
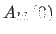
the
equilibrium magnetization of spins
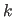
, and
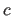
a constant.
The 3D NOE-NOE intensity can be approximated by
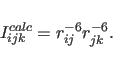 |
(20.15) |
where
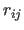
and
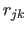
are the distances between spins
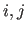
and
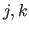
,
respectively, and the proportionality constant is set equal to one.
The 3D NOE-NOE function is given by
 |
(20.16) |
where
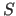
is the energy constant specified
by the SCALe statement,
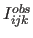
is the
observed NOE-NOE intensities,
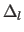
and
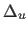
are error bounds, and
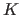
is a scale factor specified by
the RSWITch statement. The NOE-NOE connectivity
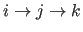
and the
intensity and error-bound information are provided by
a pair of ASSIgn statements:
The first ASSIgn statement specifies spins

and
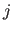
, along
with the observed intensity
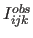
and the
error bounds
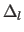
and
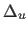
. The second ASSIgn
statement specifies spins
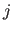
and
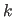
; the three real numbers
following the second selection are irrelevant and can be set
to arbitrary values.
Note: the 3D NOE-NOE function can be used in combination with
R-6 or center averaging. For further details, the reader is referred
to
Habazettl et al.
(
1992a,
b)
and
Bernstein et al. (1993).
Xplor-NIH 2025-03-21
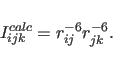
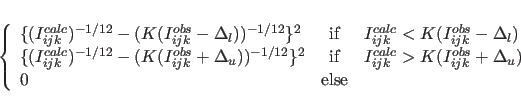
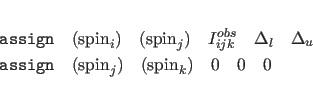

![\begin{displaymath}
I_{ijk}(\tau_{m1},\tau_{m2}) = c[exp(-{\bf R}\tau_{m2})]_{ij}
[\exp(-{\bf R} \tau_{m1})]_{jk}A_{kk}(0),
\end{displaymath}](img664.png)
